Develop the playing skills necessary for performing octaves along and across the guitar fingerboard. The technique was made famous by guitarists such as, “Wes Montgomery,” and “George Benson,” along with “Jimi Hendrix.” This lesson runs through the details behind how to achieve this interesting guitar technique…
Q: How Can I Master Octaves on Guitar?
There is a guitar soloing technique called Octaves that I know Santatna, Hendrix and Wes Montgomery have all used in their music. My problem is I cant seem to get Octaves to work in my own playing. Can you do a lesson about octaves? I would like to know the best shapes and a few ways that they can be used for playing basic melody, as well as, during improvised soloing work.
– Bryce Minneapolis, MN. U.S.A.
A: Thanks for writing in. Octaves are a very cool technique that will really beef up the tone of a melody line, (compared to only playing on a single-note). Once we add in an octave we not only generate a second note of the same tonal name, but we get the added punch of two pitches on different diameter string sets on the guitar. It is really this thickening of the sound that sets the octave effect apart from practically any other interval combination we can establish on the neck.
POPULAR OCTAVE SHAPES:
The best way to think about the most popular octave shapes on the guitar neck is to think about their roots being located on the 6th, 5th, 4th and 3rd strings. Each string will have a specific octave layout. Once the shape for that particular string set has been memorized and it is comfortable to play, it will be easy to quickly move them around the guitar neck.
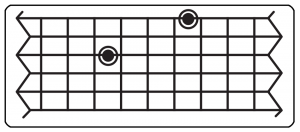
Shape #1). 6th-String Root
NOTE: Shape #1 off of the sixth string is the same geometrical pattern as the shape found off of the fifth string.
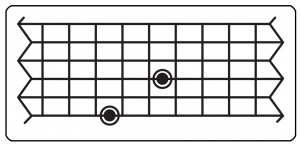
Shape #2). 5th-String Root
When we take the octave shape to the fourth string we see a change in the geometry of the shapes layout. This is because the 3rd string to the 2nd string is tuned with the interval of a major third and the other strings are tuned to an interval of a perfect fourth.
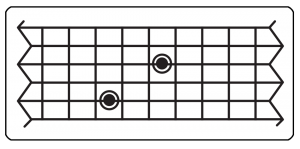
Shape #3). 4th-String Root
NOTE: The octave shape that is based off of 3rd-string is the same as the shape off of 4th-string.
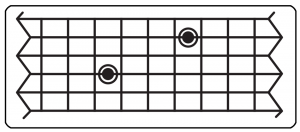
Shape #4). 3rd-String Root